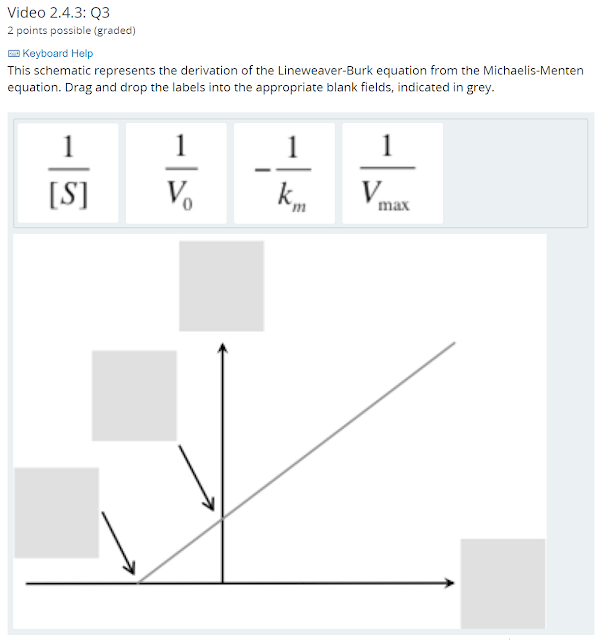
v0=vmax[S]km+[S]1v0=km+[S]vmax[S]1v0=kmvmax[S]+[S]vmax[S]1v0=(kmvmax)(1[S])+1vmax
It is easier to fit a line than to fit a hyperbola. This plot allow us to get more accurate estimate of km and Vmax, and also to plot kinetic data as a line instead of a hyperbolic curve.
Note that while we can visualize 1Vmax and 1km on the line, it is not counted as "directly" visualize Vmax and km, therefore the first option is not correct.
The x axis is 1[S]
The y axis is 1V0
The y-intercept is 1Vmax
The x-intercept is −1Km
Vmax=13×107=3.33×10−8=33.33×10−9molLmin.
Vmax=15×104=2×10−5=20×10−6molL.



No comments:
Post a Comment