Problem:
Solution:
There must be a lot of different ways to approach this problem, but personally, I like to address geometric problem analytically, or even better, algebraically.
First, we recall the fact that an inscribed circle's center is at the intersections of the angle bisectors. The equation of the angle bisector for the right angle is particularly easy, it is simply $ y = x $, now if we found another angle bisector's equation, then we are done.
Suppose we draw the angle bisector of the angle between the hypotenuse and the base, we get a diagram like this.
I want to find the y-intercept value $ d $ of that angle bisector, because then I can use intercept form for straight-line to find the equation of it. Now we know that $ \frac{d}{3} = \tan\frac{\alpha}{2} $, now we can use this awesome formula I found online.
$ \tan{\frac{\alpha}{2}} = \frac{1-\cos\alpha}{\sin\alpha} $
Now we can easily calculate $ d = 3\tan{\frac{\alpha}{2}} = 3\frac{1-\cos\alpha}{\sin\alpha} = 3\frac{1 - \frac{3}{5}}{\frac{4}{5}} = \frac{3}{2} $.
Therefore, the angle bisector's equation is $ \frac{x}{3} + \frac{y}{\frac{3}{2}} = 1 $, in intercept form.
Solving the intersection point of this with $ y = x $ gives $ x = y = 1 $, that is the center of our inscribed circle, and therefore the radius is also 1.
So the answer is (A) - A for appetizer?
Solution:
There must be a lot of different ways to approach this problem, but personally, I like to address geometric problem analytically, or even better, algebraically.
First, we recall the fact that an inscribed circle's center is at the intersections of the angle bisectors. The equation of the angle bisector for the right angle is particularly easy, it is simply $ y = x $, now if we found another angle bisector's equation, then we are done.
Suppose we draw the angle bisector of the angle between the hypotenuse and the base, we get a diagram like this.
I want to find the y-intercept value $ d $ of that angle bisector, because then I can use intercept form for straight-line to find the equation of it. Now we know that $ \frac{d}{3} = \tan\frac{\alpha}{2} $, now we can use this awesome formula I found online.
$ \tan{\frac{\alpha}{2}} = \frac{1-\cos\alpha}{\sin\alpha} $
Now we can easily calculate $ d = 3\tan{\frac{\alpha}{2}} = 3\frac{1-\cos\alpha}{\sin\alpha} = 3\frac{1 - \frac{3}{5}}{\frac{4}{5}} = \frac{3}{2} $.
Therefore, the angle bisector's equation is $ \frac{x}{3} + \frac{y}{\frac{3}{2}} = 1 $, in intercept form.
Solving the intersection point of this with $ y = x $ gives $ x = y = 1 $, that is the center of our inscribed circle, and therefore the radius is also 1.
So the answer is (A) - A for appetizer?
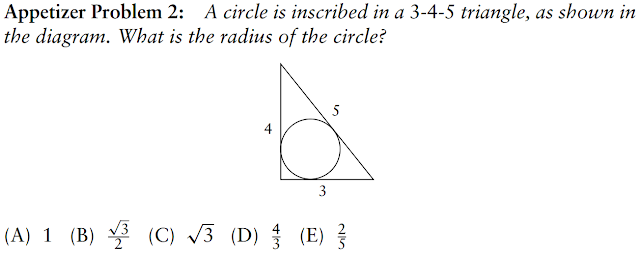

No comments:
Post a Comment