Problem:
Solution:
Fun problem for the morning.
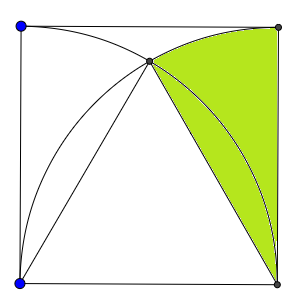
First, our goal is the area on the side:
The equilateral triangle has area is $ \frac{1}{2}a^2 \sin \frac{\pi}{3} = \frac{\sqrt{3}}{4}a^2 $
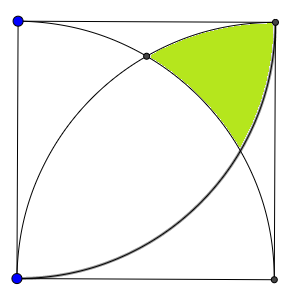
The sector have area $ \frac{\frac{pi}{6}}{2\pi}\pi a^2 = \frac{1}{12}\pi a^2 $
Therefore the remaining area on the side is $ a^2 - \frac{\sqrt{3}}{4}a^2 - 2(\frac{1}{12}\pi a^2) = a^2(1 - \frac{\sqrt{3}}{4} - \frac{\pi}{6}) $
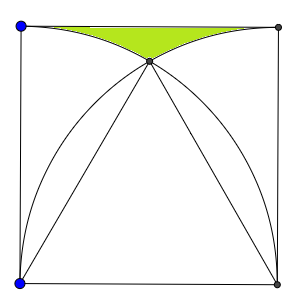
Next, we want the area of the petal.
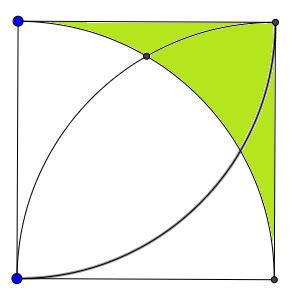
The remaining area on the corner is $ a^2 - \frac{1}{4}\pi a^2 = a^2(1 - \frac{\pi}{4}) $
Therefore the petal area is $ a^2(1 - \frac{\pi}{4}) - 2(a^2(1 - \frac{\sqrt{3}}{4} - \frac{\pi}{6})) = a^2(\frac{\sqrt{3}}{2} + \frac{\pi}{12} - 1) $
Finally, the area we wanted is $ a^2 - 2a^2(1 - \frac{\pi}{4}) - 2a^2(\frac{\sqrt{3}}{2} + \frac{\pi}{12} - 1) = a^2(1 - \sqrt{3} + \frac{\pi}{3}) $
Solution:
Fun problem for the morning.
First, our goal is the area on the side:
The equilateral triangle has area is $ \frac{1}{2}a^2 \sin \frac{\pi}{3} = \frac{\sqrt{3}}{4}a^2 $
The sector have area $ \frac{\frac{pi}{6}}{2\pi}\pi a^2 = \frac{1}{12}\pi a^2 $
Therefore the remaining area on the side is $ a^2 - \frac{\sqrt{3}}{4}a^2 - 2(\frac{1}{12}\pi a^2) = a^2(1 - \frac{\sqrt{3}}{4} - \frac{\pi}{6}) $
Next, we want the area of the petal.
The remaining area on the corner is $ a^2 - \frac{1}{4}\pi a^2 = a^2(1 - \frac{\pi}{4}) $
Therefore the petal area is $ a^2(1 - \frac{\pi}{4}) - 2(a^2(1 - \frac{\sqrt{3}}{4} - \frac{\pi}{6})) = a^2(\frac{\sqrt{3}}{2} + \frac{\pi}{12} - 1) $
Finally, the area we wanted is $ a^2 - 2a^2(1 - \frac{\pi}{4}) - 2a^2(\frac{\sqrt{3}}{2} + \frac{\pi}{12} - 1) = a^2(1 - \sqrt{3} + \frac{\pi}{3}) $


No comments:
Post a Comment